今天我们给出第八组袋鼠竞赛的题目（每个年级组一道题），并给出第七组题目（见《袋鼠数学竞赛题选（七）》）的解答。这也是我们近期的袋鼠竞赛系列的最后一组题，下一篇文章将提供题目的解答，但不会给出新的题目。

1-2年级组

The numbers 1, 5, 8, 9, 10, 12 and 15 are distributed into groups with one or more numbers. The sum of the numbers in each group is the same. What is the largest number of groups?

把1，5，8，9，10，12，15这几个数分成若干组，使每组数的和相等。最多可以把这些数分成多少组？

3-4年级组

Karin wants to place five bowls on a table in order of their weight. She has already placed Q, R, S and T in order. Bowl T weighs the most. Where must she place bowl Z?

Karin想把5个碗按照重量排列在桌上。她已经把Q，R，S，T四个碗排好了，其中T是最重的碗。她应该把碗Z放在哪个位置上？

5-6年级组

Five squirrels A, B, C, D, and E are sitting on the line. They pick 6 nuts marked by crosses. At one moment the squirrels start running to the nearest nut at the same speed. As soon as a squirrel picks a nut it starts running to the next closest nut. Which squirrel will get two nuts?

7-8年级组

A 3 cm wide rectangular strip of paper is grey on one side and white on the other. Maria folds the strip, as shown. The grey trapeziums are identical. What is the length of the original strip?

--------   分割线   --------

下面是第七组题目的参考答案和解析。

1-2年级组

Which pattern can we make using all five cards given below?

使用所给的5张卡片，可以摆出哪个选项中的图案？

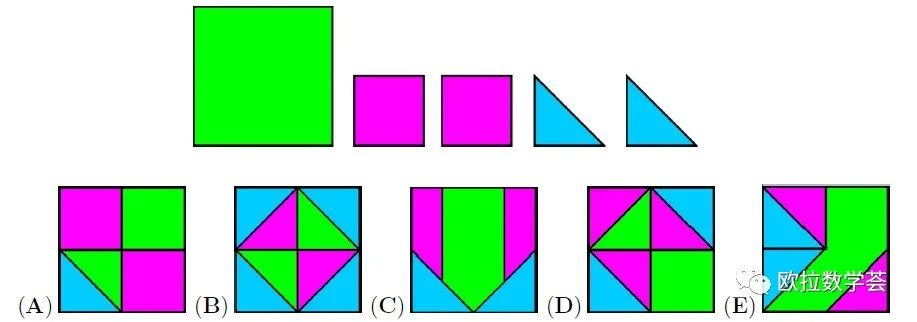
【答案：A】显然，卡片之间会有交叠遮盖的效果。选项（B）中，蓝色的面积比蓝色卡片的总面积大，所以不可能。选项（C）中，粉色部分不可能由两张粉色卡片拼出来。选项（D）类似于（C）的理由。选项（E）中，右下角的粉色区域不可能构造出来。

3-4年级组

Magic trees grow in a magic garden. Each tree contains either 6 pears and 3 apples or 8 pears and 4 apples. There are 25 apples in the garden. How many pears are there in the garden?

魔术花园里种了一些魔法树。每棵树上要么结6个梨子和3个苹果，要么结8个梨子和4个苹果。已知花园里共有25个苹果，那么花园里共有多少个梨子？

【答案：D】两种树的数量不确定，有多种可能性。但无论是哪种树，树上的梨子数量都是苹果数量的2倍。所以梨子的总数也一定是苹果的2倍。

5-6年级组

The number 2581953764 is written on a strip of paper. John cuts the strip 2 times and gets 3 numbers. Then he adds these 3 numbers. Which is the smallest possible sum he can get?

一张纸条上写了一个数2581953764。John把纸条剪了两刀，剪成3截。他把三截纸条上的三个数相加，所得的和数最小可能是多少？

【答案：B】首先，要使和数的位数尽量少。因为三个数的总位数是10位，所以最大的加数至少是四位数。其次，要使四位数的加数的千位数字最小。所以首先应该考虑选择1953作为四位数的加数。此时，另外两个加数分别为258和764，三个数的和是2975。如果要使和数更小，四位数的加数千位数字不能大于2，对应的能得到最小和数的切分结果是（2581，953，764），它们的和显然大于2975。

7-8年级组

Ivor writes down the results of the quarter-finals, the semi-finals and the final of a knock-out tournament. The results are (not necessarily in this order): Bart beat Antony, Carl beat Damien, Glen beat Henry, Glen beat Carl, Carl beat Bart, Ed beat Fred and Glen beat Ed. Which pair played in the final?

Ivor记录了一场淘汰赛的四分之一决赛、半决赛和决赛的结果。他记录的结果如下（不一定按照比赛顺序）：Bart战胜Antony，Carl战胜Damien，Glen战胜Henry，Glen战胜Carl，Carl战胜Bart，Ed战胜Fred，Glen战胜Ed。请问，哪两个人在决赛中对阵？

【答案：B】根据淘汰赛的规则，只要输一场比赛就不能继续参加后面的比赛了。所以，要打入决赛，必须在四分之一决赛和半决赛获胜。虽然题目中没有告诉我们哪些场次是什么类型的比赛，但我们可以统计获胜的场次。在所有7场比赛中，Glen获胜3次，Carl获胜2次，Bart和Ed获胜一次。所以应该是Glen和Carl进入决赛，并且是Glen获得冠军。